Probability (P1 Exam Practice) - Sample Exam Problems from theinfiniteactuary.com
Thought I would use my old saved resources to study for this test seeing that it is pretty hard. You need to know all of your calculus, a lot with the exponential function which is the easiest to know. They also expect you to know how to take integrals using integration by parts (usually calculating the integral of xe^x). Here are some useful questions I found.
The only problem with theinfiniteactuary.com is that the person that wrote out the answers to the problems was sloppy, barely an explanation, and didn't use proper notation. I will try my best to make it more understandable.
Example 1
A life insurance company classifies its customers as being either high risk or low risk. If 20% of the customers are high risk, and high risk customers are three times as likely as low risk customers to file a claim, what percentage of claims that are filed come from high risk customers?
First, ask what they gave us. See that it says high risk customers are three times as likely as low risk customers to file a claim, so we have...
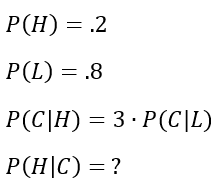
We also have an equation that we can manipulate, or prove what we have is useful.
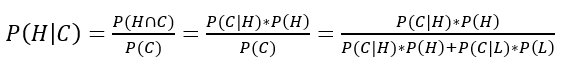
Now, we can solve the equation because we have everything and some stuff cancels out.
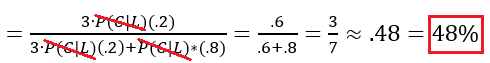
Example 2
Suppose we have (X,Y) is uniformly chosen from the set given by  . Find the marginal density function
. Find the marginal density function 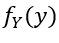 of Y.
of Y.
It has been made especially clear to me that you need to graph (by hand) all of the boundaries they give you. Since it is uniform we can find a height (or density) to our graph by finding the area in the bounded region.
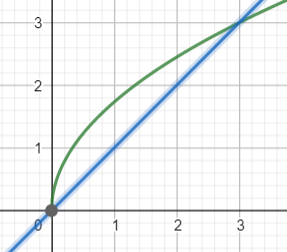
Take the integral of the top curve minus the bottom like this:

This means our density is 2/3. The question says to find the marginal density function of Y. So, we throw out the x integral and change the bounds of the y integral using the equation  . The integral becomes...
. The integral becomes...
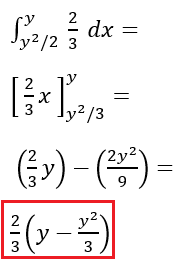
Example 3
If X is a Poisson random variable with P[X = 1] = 2.5P[X = 0], then what is the probability that X will be within 1 standard deviation of EX?
First, know that Poisson Distribution functions can be set equal to find lambda  .
.
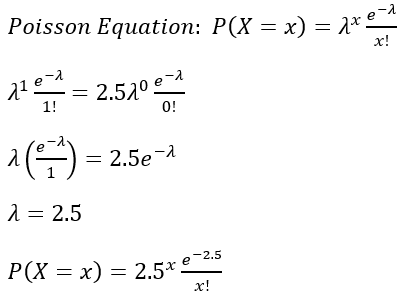
By some of the rules you memorize (or can prove), we know
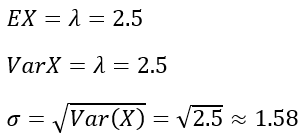
Since Poisson is discrete we only look at the integers in the range given. Plug in and calculate for the answer.
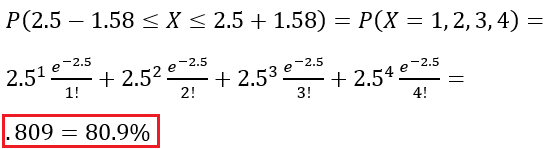
Educational Archives
Option Volatility and Pricing
1, 2, 3, 4, 5, 6, 7-1, 7-2, 7-3
Actuarial Mathematics
Physics
Motion in 2-Dimensions, Kinematics, Kinematics Quiz & Solutions, Forces in 1-Dimension, Forces in 2 - Dimensions, Forces 1 & 2 Dimensions, Kinematics & Forces Review, Kinematics & Forces Quiz & Solutions, Kinematics & Energy Quiz & Solutions, Momentum, Circular Motion, Circular Motion & Gravity, Midterm Exam, Midterm Solutions
SAT Math
Asymptotes, Composite Functions, Contest 1 - Area, Contest 1 – Winners and Solution, PEMDAS, Systems of Equations, Functions, Exponents, Contest 2 - More Functions, Contest 2 – Winners and Solution, Percents, The Interest Equation, All About Division, Contest 3 - Factoring, Contest 3 – Winners and Solution, Fractional and Negative Exponents, Basic Trig, Contest 4 - Math Vocab, Trig 3, Contest 5 – Winners and Solution, Contest 6, Contest 6 – Winners and Solution, Functions, The Unit Circle, Compound Inequalities, Composite Functions
Puzzles


Ugh math good thing I don’t have to take it this year
This is quite a bit harder that your math class last year. Haha.
Yep that’s true
Have you tried weku yet