Probability (P1 Exam Practice) - Binomial and Continuous Uniform Distribution
Actuary Exam P1 Practice
Well... Didn't quite pass the exam the first time, but got some valuable insight on what to expect next time. The P1 usually gives harder examples, a lot of Poisson and Joint Probability distributions. Next time I guess. Haha. Enjoy today's lesson!
Binomial Distribution
The binomial distribution depends on successes (p) and failures (q). With a given probability of success or failure and the number of trials we can find the probability of anything we want to know about the problem. These problems require combinations because arrangement of successes and failures does not matter. If you want to succeed 4 times out of 10 there are 10 choose 4 ways to do that or 10! / ( 6! * 4! ), then just multiply that times the number of p's and q's.
Equation
Example
Ben is making shots at a basketball hoop from the freethrow line. He makes about 35% of the shots.
If he shoots 10 times, what is the probability that he makes 4 shots?

If he shoots 15 times, what is the probability that he makes between 9 and 12 shots (inclusive).
All we have to do here is add the probabilities of 9, 10, 11, and 12.
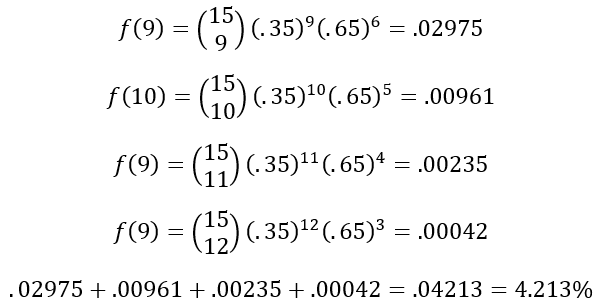
Uniform Continuous Distribution
This distribution is used when you have in interval or range of events that all have the same probability. On a graph it just looks like a flat line from two numbers A to B. If you know what you interval is (usually given in problems) then you can find everything you need to know including mean, variance, probabilities.
Equations
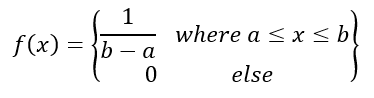
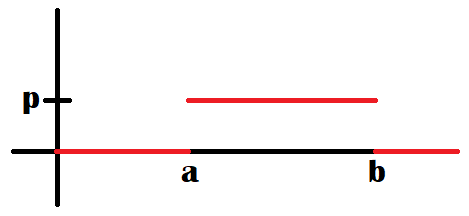
a is the lower bound and b is the upper bound.
Example
Find the pdf and df for a uniform distribution over the interval [ 6 , 10 ].
Graph & Solution

We need to find p, so to do that we plug our givens into the equation above.
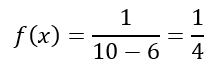
Then we integrate with some calculus (which there is a lot of on the P1 Exam) to find our df.
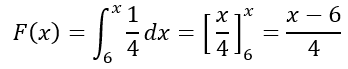



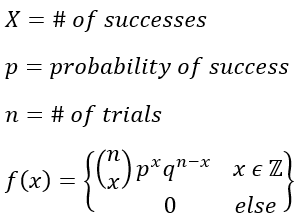
How’s it going are you doing good
Yeah. I'm living the life right now. Haha. Going to need to find a job soon. Money needs to be made. How about you?
Using dtube and earning more