THE SOLID ANGLE - PART 2 - BASIC BACKGROUND (CONTINUATION)
Regards Steemians!. The present post is the second of a series dedicated to showing in a clear and simple way the definition of SOLID ANGLE, presenting the necessary equations and mathematical definitions. The first can be found in:
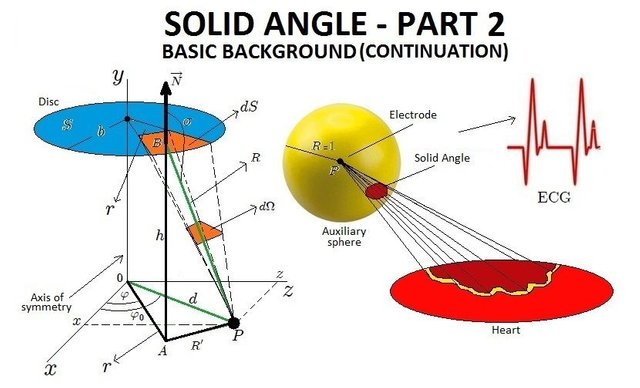
Continuing with the basic background for a good understanding of the definition of Solid Angle and how it is calculated, in this post I will talk about the Dihedral Angle, the Polyhedral Angle and the intersection of a polyhedral angle with a sphere.
THE DIHEDRAL ANGLE
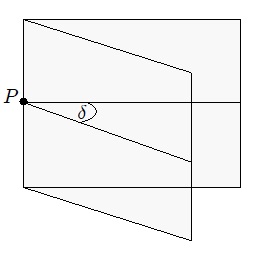
| A Dihedral Angle is that formed by the intersection of two planes, as shown in Figure 1. |
|---|
THE POLYHEDRAL ANGLE
| A Polyhedral Angle is the part of the space bounded by several non-coplanar plane angles, with common vertex V and shared sides (each side is common to two angles) as, for example, the polyhedral angle shown in figure 2. |
|---|


- The Convex: is the polyhedral angle that remains in the same half-space with respect to the planes of each of its faces.
- The Concave: is the polyhedral angle in which when extending the plane of one of its faces, a part of the angle is in a half-space and the rest in the other.
INTERSECTION OF A POLYHEDRAL ANGLE WITH A SPHERE
Before continuing, it is necessary to define The homothety: is the transformation that makes correspond each point A of a figure another point A' (homologous of A) aligned with A and with center of homothety 0, so that:
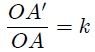
Here k is a non-zero constant, called Homothety Ratio.

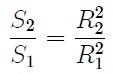
It is possible to find the surface S₁ as a function of only the surface S₂ and the radius R₂ of the sphere that defines this last surface. In effect, when doing 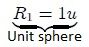 (u is a unit of measure) in equation 2 you get,
(u is a unit of measure) in equation 2 you get,
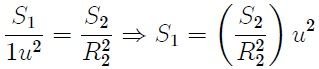

where  is the surface determined by the polyhedron angle on the surface of the unit sphere and S is the surface determined by the same angle on the sphere of radius R. Note that the quantity
is the surface determined by the polyhedron angle on the surface of the unit sphere and S is the surface determined by the same angle on the sphere of radius R. Note that the quantity  is dimensionless and numerically equal to
is dimensionless and numerically equal to  . If this amount is called Ω then,
. If this amount is called Ω then,
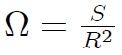
which is the key equation for the mathematical definition of solid angle.
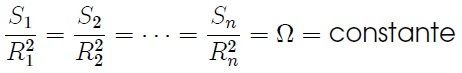
REFERENCES
- Soldovieri, Terenzio & Viloria, Tony. EL ANGULO SOLIDO Y ALGUNAS DE SUS APLICACIONES. 1era edición (borrador). You can download it on my website http://www.cmc.org.ve/tsweb/
- All the images presented here were elaborated by me. The color image constitutes a modification of the cover image of the text indicated above, of which i'm the author..
- Moya de la T. D., A. PRINCIPIOS DE GEOMETRIA. Imprenta de D. Alejandro Gómez
Fuentenebro, 2da edition, 1864. pp. 67. - Alvarez C., E. ELEMENTOS DE GEOMETRIA, CON NUMEROSOS EJERCICIOS Y GEOMETRIA DEL COMPAS. Editorial Universidad de Medellín, 2003. pp. 368 - 370.
- Izquierdo A., F. GEOMETRIA DESCRIPTIVA. Editorial Paraninfo, 24a edition, 1998. pp.
68 - 76. - Zuñiga P., M. L. POLIEDROS ARQUIMEDIANOS. Revista del Profesor de Matemáticas,
(6):49 – 57, 1998. - Puig A., P. CURSO DE GEOMETRIA METRICA - FUNDAMENTOS, volume 1. Biblioteca
Matemática S. L., Madrid - España, 11a edition, 1973. pp. 117 - 128. - Faget, J. & Mazzaschi, J. TEMAS PROGRAMADOS DE FISICA - GENERALIDADES, volumen 1. Editorial Reverté, S.A., 1976.

It is my wish that the present information can be very useful to all. The next in this series will refer to the Surface and its vector representation.
Sorry for my English!.
Until my next post. Regards! 😁


Nice explanation, but a little bit difficult to understand :-)
Thanks @asgharali. 😉
This is really some interesting stuff :)
Thanks @deepeshgarg. Regards! 😁
Thanks @steemiteducation. I'm already following you. I'll be attentive to your blog posts. Regards!.
Muy esmerada la presentación de este trabajo. Felicitaciones ( ahora entiendo por qué te desapareces)
Me descubrió!!! mi muy hermosa colega @emily61 jajajajajajaja 😈 😁
Great post! Congratulations.
Thanks my friend @rnunez09. Regards!.
Congratulations brother @tsoldovieri, excellent post with great presentation. Greetings!
Thanks my perijanero brother @hugobohor. Regards!.