Beyond the standard model : Holographic Principle Could Hold In Our Universe

amity123 (61)in #steemstem • 7 years ago

All we wanted to know about the end of the world, but were afraid to ask

From where we stand, the universe looks three-dimensional. But a major theory of theoretical physics suggests that it takes one less dimension to describe the universe.

Compactified measurements are good in the short term, but they are not ideal for the universe in the long run. "Now we seem to be standing on the top of the building, but have not yet jumped off of it. If we do not do it of our own free will, quantum mechanics will do it for us, "dropping" to the lowest level of the energy state. "The reason why the universe with ten non-compactified dimensions is ergonomically preferable is that in the most modern models , known today, the energy of the vacuum is a consequence of the compactification of additional dimensions.In other words, the dark energy, about which so much is said, does not simply control the accelerating expansion of the universe, but a part ie all) of the energy goes into the hold of extra dimensions compactified in a state compressed tighter than a spring of Swiss watches. Only in our universe, as opposed to hours, "Roleh", and it makes the flow of battle.

In other words, the system in question has a positive potential energy. The smaller the radius of additional measurements, the tightened the spring and the greater the stored energy. Conversely, as the radius of these measurements increases, the potential energy decreases, reaching zero when the radius becomes infinite. This is the lowest level of energy state and,
consequently, a really stable vacuum, a point at which the density of dark energy reaches zero, and the radii of all ten dimensions become infinitely large. In other words, once small internal measurements become decompactified.

Decompactification is the reverse of the compactification phenomenon, which, as we have said, constitutes one of the biggest problems in string theory: if this theory describes the Universe as a ten-dimensional one, why do we see only four dimensions? String theorists find it difficult to answer the question of how the additional dimensions of the theory are so carefully hidden, since, in the opinion
Linde, other things being equal, the measurements should be fairly large. This is similar to trying to increase the volume of water in a filled reservoir with rigid walls. In every direction, in any corner of such a structure, water will tend to the outside. And she will not stop her attempts until she realizes them. When this happens and the walls of the tank suddenly succumb, the water limited by a compact area (the volume of the reservoir) will pour out and spread over a vast surface. Based on the current understanding of string theory, the same will happen with compact measurements - folded into Calabi-Yau spaces or other complex configurations. Regardless of which configuration is selected for internal measurements, they will eventually unfold and unfold.

The Calabi-Yau manifold

Of course, the question may arise: if the decompaction of measurements is beneficial from the energy point of view, then why has not this happened so far? One of the solutions offered by physicists attracts branes and streams. Suppose that we have a pumped-up bicycle camera, which can cause a blister in some of the thinnest places of a bicycle tire - a bubble that eventually breaks. You can strengthen the weak spot of the tire by applying a patch that resembles a brane, or rewind the whole tire with a rubber band to keep it in shape, which is done by flows with the Calabi-Yau spaces. That is, the essence of the idea is that we have two opposite forces - a natural desire to expand (to the pumped chamber) and branes, streams and other structures that wrap around the object and, thus, hold it back.

At present, these opposing forces are perfectly balanced and in balance. However, this world is unstable. If the radius of the additional measurement increases due to small quantum fluctuations, branes and flows provide a restoring force that quickly brings the radii of measurements to the original state. But if the measurement is excessively stretched, branes or streams can burst. Giddings explains this in the following way: "in the end, a particularly strong fluctuation will increase the radius to the threshold value of decompactification" and, having appeared on the right slope of the graph (Figure 1), we will roll down to infinity.

In Fig. 2 shows a similar situation, but with one nuance. Instead of direct tunneling into the Universe with ten decompactified measurements, we will make one or several stops along the way. But in any case, whether you will fly without an intermediate landing or make a transfer in Dallas or Chicago, the final point is the same. And inevitably. And it seems that the landing will not be very soft. It is worth remembering that a change, when it happens, will be a phase transition of the vacuum, and not a ball rolling out of the pit or punching the wall. Change begins with the appearance of a tiny bubble, but this bubble will grow exponentially. Inside the bubble, the compactification of six dimensions of almost Planckian dimensions will begin to untwist itself. As the bubble grows, the space-time of the four large dimensions and six minimized measurements begin to merge. Where dimensions were divided into compactified and extended, there will be ten decompactified, combined together, dimensions without barriers separating them.

Fig 1. above. One theory claims that our universe is in a small hole on the left side of the graph, which keeps the potential energy of the vacuum (V) at a certain level, and also fixes the radius (R) of additional compactified measurements. However, such a state can not be eternal. A small perturbation can push us to the right vertex, or we can pass through the barrier through quantum tunneling and roll down the right side of the graph to infinitely large additional dimensions. This process, through which earlier tiny dimensions unfold to turn into huge ones, is called decompactification (adapted, with permission, from Steve Giddings' drawing)

Fig2. above. Here the situation is much more interesting than in Fig. 1. Our universe is still heading for decompactification and a field of infinitely large additional dimensions, only this time we are going to make an intermediate stop on the landscape along this route. In this scenario, our universe can be regarded as a small ball, which when slid down the hill temporarily lingered in the hollow (A). In principle, the ball could make a lot of time stops when descending, although this chart shows only one additional cavity (B) (adapted, with permission, from the Steve Giddings picture)

"We're talking about a bubble that expands at the speed of light," says Shamit Kachra. - It occurs at a specific place in space-time, similar to the birth of bubbles in the water. The difference is that such a bubble does not rise immediately upwards and does not burst. On the contrary, it expands and displaces all water. " But how can a bubble move so fast? The reason is that the decompactification state inside the bubble is at a lower energy level than the world outside it. Since the systems naturally evolve into states with lower energy, in our case, in the direction of increasing the radius of compactified measurements, the gradient of potential energy creates a force at the edge of the bubble, causing acceleration directed outward.

This acceleration accelerates the bubble to the speed of light in a fraction of a second. Linde describes this phenomenon in a more colorful way: "The bubble tends to move as quickly as possible, and if you can perfectly
exist at a lower energy level, then why wait? He asks. "Therefore, the bubble moves faster and faster, but it can not move faster than light." 10 Although, if he knew what kind of reward awaits him, he would try to overtake the world if he could. Due to the fact that the bubble grows at the speed of light, we will never know what will happen to us. The only preliminary warning that we will receive is a shock wave that will come earlier for a split second. A bubble with tremendous kinetic energy will be attacked by the front of this shock wave. But this is only the first round of the end of the world.

Since the wall of the bubble has a small thickness, it will take a fraction of a second to make the worst happen. In a place that we call our home, the four-dimensional laws of physics act, while the inner part of the bubble obeys the laws of a ten-dimensional space. And these laws will come into effect as soon as the inner part of the bubble penetrates into our world. As the playwright David Mamet once remarked: "Everything changes."

But by all indications, pressing the alarm button is still too early. It can take an extremely long time (approximately e ^ 10 ^ 20)) years before the disintegration of our space-time. This number is so large that it is difficult to imagine even mathematicians. We are talking about one of the fundamental constants of nature, a number that is approximately 2.718, multiplied by itself 10 ^ 120 (one unit with one hundred and twenty zeros) times. If this rough assumption is true, then our waiting period will be almost infinitely long.

Where, then, did the number e ^ (10 ^ 120) take in our estimate? The premise is that our Universe evolves into something called the de Sitter space, a space with a predominantly positive cosmological constant in which all matter and radiation eventually become so sparse that they practically disappear. For the first time such a space was described in 1917 by the Danish astrophysicist Willem de Sitter as a solution to Einstein's equations for empty space. If our universe with its small cosmological constant is "de Sitterova", then the entropy of such a space will be very large, of the order of 10 ^ 120 (from which this estimate was taken a little later). Entropy is so great, because the volume of the de Sitter universe is large. Just as in a large box there are more different places in which an electron can be placed than in a small one, such a large universe has more possible states (and consequently, a higher entropy) than a small one.

The de Sitter space has a horizon of events, as well as a black hole. If you get closer to the black hole and cross the rockcracker, it will drag you inside, and you will not return home for dinner. The same is true for light that can not leave the hole. This is also true in the case of the de Sitter horizon. If you go too far into a space that expands with acceleration, then you can never return to the point where you started. Light, as in the case of a black hole, also can not go back.

When the cosmological constant is small, and the accelerated expansion occurs relatively slowly, as is the case in the modern world, the horizon is very far away. That's why the volume of this space is so big. Conversely, if the cosmological constant is high, and the universe expands at a tremendous rate, then the horizon (or critical point) can be very close - almost at hand (literally), and the volume will accordingly be small. "If you shove your hand too far into such a space," explains Linda, "then a rapid expansion can tear you away from it."

Suppose that there is a potential minimum at point A and a separate minimum at point B, where point A is located higher than B, and therefore has a greater vacuum energy. Since the point A has a higher energy, then gravity is stronger, and the space in it will have a higher curvature. And if we treat this space as a sphere, its radius will be smaller, because spheres of smaller dimensions have greater curvature than larger spheres. Since the point B has a lower energy, gravity will be weaker. Consequently, the space around it will have less curvature. And if we treat this space as a sphere, its radius will be greater, and therefore it will have less curvature. We illustrated some aspects of this idea in Fig. 3 (using boxes for A and B, not spheres) to show that the object most likely travels down to the landscape with lower energies - from A to B, than upwards. For greater clarity, you can connect the two boxes with a thin tube. These two boxes eventually come to equilibrium and will have the same concentration or density of gases, and the number of molecules passing from A to B will be equal to the number of molecules passing from B to A.

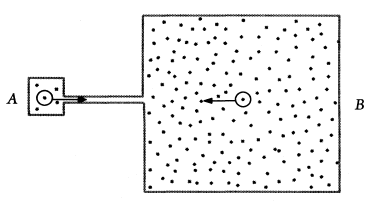
Fig. 3. This figure attempts to show why it is easier to "tunnel down" from A to B (see Figure 2) rather than "tunnel up" from B to A. The analogy presented here shows that it is more likely to detect a molecule in B , than in A, simply because the number of molecules in B is much larger than in A.

However, since B is much larger than A, it has many more molecules. Therefore, the probability of the transition of any single molecule from A to B is much greater than the probability of the transition of an individual molecule from D to A. Similarly, the probability of the appearance of a bubble that takes you to a place with low energy in the landscape is significantly higher than the probability of the bubble emerging you in the opposite direction (uphill), as any molecule is more likely to make the transition from box A to B.

In my opinion, the deployment of hidden measurements can be the most impressive spectacle ever seen, if it can, of course, be observed, although this looks extremely problematic. Let me still fantasize a little and assume that such a scenario will eventually be realized and that the great disintegration of space-time will occur at some point in the distant future. In this case, it will be a perfect confirmation (albeit belated) of the idea to which I devoted the best part of my career.

It is a pity that when the most hidden places of the universe are finally opened, and the cosmos fully reveals its multidimensional magnificence, there will be no one near to be able to appreciate it. And if someone survives the great transformation, then there will be no photons that would allow this to be observed. There will be no one who can celebrate the success of this theory, invented by creatures who called themselves humans, in an era known as the 20th century, although it is more correct to refer it to the 137,000,000th century since the Big Bang.

This perspective especially frightens people like me who spent decades solving the problem of the geometry of six internal dimensions and explaining it to people (which is even more difficult), which find all these concepts abstruse, if not absurd. Since at that moment in the history of the universe - the moment of the great cosmic "unwinding", the extra dimensions that are deeply hidden now will no longer be a mathematical abstraction, they will cease to be additional. On the contrary, they will be a legitimate part of the new order, in which all ten dimensions will exist on an equal footing, but you will never know which of them was once collapsed and which one is unfolded. You will not care. With ten dimensions of space-time and six new directions, life will get so many opportunities that we can not even imagine them.