Quantum Bonding : a new model of Atom
During the last two decades, scientists have attempted to use the unusual properties of the quantum microcosm to make a leap in the field of information processing and communications. Using some features of physics that manifest themselves at the smallest scales of nature (the fact that an electron is simultaneously a particle and a wave that an object can be in several places at the same time and that two particles can retain a ghostly instantaneous connection even if they are distant from each other for significant distances), quantum machines could make unthinkable calculations, means of communication and methods of measuring routine. Let's give just one example: a quantum computer is likely to be able to crack codes that can not be deciphered.
At the same time, quantum machines can be used to store and transmit information in such a way that its secrecy is guaranteed by the laws of physics. They can also be used to model processes in complex chemical and physical systems that can not be traced in any other way. Quantum systems, apparently, will repeatedly increase the accuracy of the world's most accurate chronometers-atomic clocks-and serve as miniature high-precision sensors for measuring the properties of chemical and biological systems at the atomic or molecular level with applications from biology and materials science to medicine.
It is because of the huge potential of such technological monsters as Google and Intel, a number of start-up companies, the Ministry of Defense and other US government agencies are making a big bet on developments in this area. Equally encouraging is the American academic community: only in 2015, three leading journals published more than 3,000 scientific articles that mention quantum computing or quantum information.
The problem is that scientists can not yet build a large quantum machine that would realize these hopes. The main difficulty is that such a computer by definition must work in the realm of the microworld, and at the same time, when we try to make a quantum computer large enough to work with it, its natural tendency manifests itself - it begins to obey the classical laws of the macrocosm .
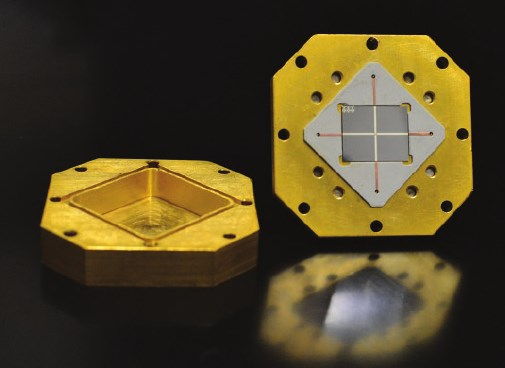
A quantum device. The electrical circuit for measuring superconducting qubits is placed in a gold-plated chamber. These measurements can quantum entangle qubits in separate clusters or modules, allowing the modules to unite with each other to form a single quantum computer.
In order to build a system that obeys quantum laws on a large scale and possesses all the power of quantum information processing, it is likely that a modular approach is required, in which smaller, obviously known quantum units are connected to each other in such a way that their quantum nature is not destroyed. In recent work, not only theoretically, but in practice, this so-called modular approach was successfully tested on a small scale, and this prepares the ground for the realization of the unique possibilities of quantum mechanics.
Probably, zeros and, possibly, units
The first assumption that the quantum world can be used to create a new type of powerful computers, in the early 1980s. physicist Richard Feynman from the California Institute of Technology and mathematician David Deutsch from Oxford University (The first idea of quantum computing in 1980 in his book "Computable and non-computable" was put forward by the Soviet mathematician Yury Ivanovich Manin.) This hypothesis remained speculative for many years, until in 1994 Peter Shor, then at AT & T Bell Laboratories, showed how a quantum computer can be used for fast factorization (prime factorization) of large numbers , which sparked a great interest in this area.
The first primitive quantum computers appeared in the late 1990's - early 2000's, when scientists built simple, consisting of several "bits" of the system, built on atoms, molecules or photons. It is the special nature of quantum particles that allows quantum computers to achieve enormous superiority over their classical counterparts. Unlike classical calculations in which the basic information unit (bit) takes a certain value - "1" or "0", the quantum information unit (qubit) can exist simultaneously in two states, that is, it can represent "0" and "1" Simultaneously.
Probably, the kbit can be "0", but possibly also "1", or with equal probability of being "0" or "1", or any other weighted combination of two binary states. Kubit has such power because a quantum particle can be in two locations or in two physical states simultaneously - a phenomenon called "superposition". In addition to the fact that qubits are in two states simultaneously, they can be related to each other by means of a quantum property called "quantum entanglement". (The English term entanglement does not have a settled single Russian equivalent, and in the literature you can find the most diverse names of this phenomenon: "quantum entanglement", "quantum nonlocality", "quantum entanglement", "quantum entanglement", "quantum linkage" etc.) Quantum entanglement is the ability of particles distant from each other in space to maintain a certain connection with each other in such a way that the action taken over one of them immediately reflects on the other. This property gives quantum computers the possibility of mass-parallel processing of information. When a set of qubits is quantum-entangled, a simple operation on one of them can affect the states of all other qubits. Even with just a few qubits, all these interdependent states "0", "1" and their superpositions form an unusually complex range of possible outcomes. While a classical computer can process only one possibility in a single clock cycle, a quantum computer can check all possible solutions to the problem simultaneously. Only a few hundred qubits can calculate a table of outcomes, the number of elements of which exceeds the number of all particles in the universe.
Until now, scientists in a number of laboratories have managed to create only small quantum computing systems with a number of qubits not exceeding 10. But as we add qubits, it becomes increasingly difficult to protect the system from the outside world - and any such impacts are disastrous for those very properties, which make quantum computers so unusual. A quantum superposition of a set of states can exist only in an isolated system. Any attempt at its premature observation or measurement will lead to their collapse into one of the possible states - to the choice of one of the possibilities. In this situation, quantum mechanics ceases to work and the qubits again turn into ordinary bits of the classical computer. In other words, the special features of quantum objects can usually be observed only in microscopic systems, and they are destroyed when these objects become completely connected to a larger whole - in a similar way to how an indie-like music group probably likes it the most To their fans, if only a few have heard of it. Large systems are usually too complex and not sufficiently isolated to behave according to quantum-mechanical laws - after all, we do not expect to find a baseball or even a biological cage in two places at the same time.
Three ways to build a quantum computer
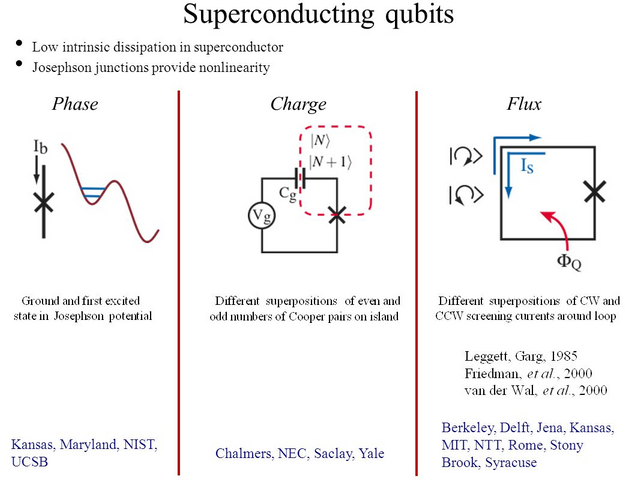
Computers that are built on the basis of strange laws of quantum mechanics, theoretically can perform calculations that are not available for classical computers. However, the more a quantum computer becomes, the more difficult it is to preserve its quantum properties. Scientists believe that solving the problem is to build a lot of small quantum computers and put them together into one larger whole: a strategy called "modular quantum computing." The boxes on the right show three potential modular circuits using three different types of quantum bits or qubits.
Quantum property 1: superposition
Atoms and subatomic particles can exist in a set of states simultaneously - a state called superposition. If a classical object, such as a billiard ball, can rotate simultaneously in only one direction, the quantum particles can be in two "spin states" - both with the spin directed upwards and with the spin directed downwards - simultaneously. Using this property, quantum computers are likely to be able to analyze many possible solutions to the problem at the same time.
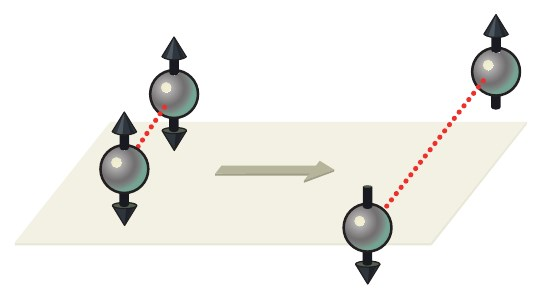
Quantum property 2: quantum entanglement
Albert Einstein called it a phantom long-range: quantum entanglement allows two particles to build such a connection that the action taken on one of them instantly affects the other, even if they are separated from each other in space. In the figure below, the quantum-entangled particles are first in a superposition of states with spin up and down. When an external dimension causes particles to "choose" a single state, both particles always come in coordinated states. Depending on the type of quantum entanglement, if the first particle is in a state with spin up, the second one will always be in a spin-down state. When a lot of qubits are entangled, the operation carried out over one will instantly affect others, opening the possibility of an unprecedented scale parallel processing of information.
Method # 1: Atomic ionic qubits
The easiest way to build a modular quantum computer is to use single atoms as qubits. Each atom can represent the value "0" or "1" of the binary code (or their superposition) through various electronic orbits (above). Below, a schematic image of three modules - quantum minicomputers consisting of five atomic ions each - connected in such a way as to preserve the quantum properties of each of the modules. Within each module, all five ions are confused with each other. Two ions at the ends (white) are special and can emit photons to communicate with other modules.
Method 2: Superconducting qubits
In another strategy for constructing a quantum computer, "artificial atoms" are used as qubits, which are superconducting circuits. These qubits are electrical circuits that can take the value "0" or "1" depending on the presence or absence of a microwave photon or alternating current flowing through the circuit. (When the qubit is in the superposition state, the photon may be there and simultaneously it may not be.) Within each module, the qubits can be quantum-entangled directly to each other by captured photons. These photons can also be sent over optical fibers to link each module to others.
Method 3: Solid-state quantum qubits
The third option is to make qubits from defects in a solid, such as a diamond crystal lattice consisting of carbon atoms. If one of the carbon atoms in the lattice is replaced by a nitrogen atom, and the place in the neighborhood is left empty, a defect called the nitrogen-vacancy center (or the NV center) will be obtained. The NV center and the surrounding carbon atoms all become qubits, and their spin states are "0" or "1". Each cluster of defects in the crystal lattice is a separate module, and the modules themselves can be connected to each other by means of optical photons.
Modular Quantum Systems
The scientists face the difficult task of scaling the system without losing its quantum nature. The use of "brute force" to build large quantum systems by simply adding new qubits and combining them into a single network will most likely fail. This prediction is reinforced by the fate of machines developed by the Canadian firm D-Wave Systems, which connected together several hundred or even thousands of individual qubits. Although company representatives claim that their devices beat records of the speed of performance of classical algorithms, we did not find any publications with data that would indicate a quantum entanglement on a large scale or about the advantages in the speed of processing information by such systems.
However, the modular methodology tells us another way to the goal. This technical solution is akin to the strategy that commercial airlines use to cope with the problems of customer service. The next time you fly an airplane, consider carefully the back cover of the advertising magazine of the airline offered to passengers on board the aircraft. The company's route map gives a rough idea of how a full-scale quantum computer might look. Airlines are not in a position to connect each city directly with each other individual airline, as logistics and overhead in this case would be prohibitive. Instead, they use central interchange nodes to organize a network of indirect messages. Sacrificing the advantages of direct communication, in return they get the opportunity to grow and serve a large network of airports.
Similarly, in a modular quantum computer, there is no connection for each qubit with each. Instead, several qubits in it will be used as the core of the network, through which separate modules will be connected, akin to how Atlanta serves as a transfer point connecting the southeast of the US with the rest of the regions. Modular networks will help keep the number of interactions between qubits in an amount that allows them to be managed successfully, and at the same time such an architecture will allow each module to remain protected from external influences. They compensate for the need to sacrifice the advantage of direct connections between qubits, giving in return the opportunity to interact through an intermediary to thousands or even to millions of qubits. But unlike traditional modular systems, such as multi-core processors of computers, in which both the same type of conductors are used for communication between processors and processors, to obtain the necessary quantum entanglement and at the same time to preserve isolation between modules, a modular quantum system , may require two or even more different types of communication. In the past decade, there were three main modular quantum strategies using different types of qubits.
Three of us independently develop these platforms, and we believe that they will get accustomed to larger quantum computers, which will open the possibility of applying new types of information processing.
Atomic qubits
The most natural type of qubit is a single atom whose electronic or nuclear energy levels (sometimes called spin states) store quantum information. Atomic qubits by their very nature are amenable to scaling, because multiple atoms of the same species are virtually identical and do not require any additional settings to resemble each other. Laser beams can cool atoms to an almost immobile state, freezing them by transferring momentum from an atom to scattered laser radiation. We learned to do all this by suspending the atoms inside the empty space of the vacuum chamber to protect them from any external influence.
Neutral or charged atom (ion) can serve as a qubit. In order to keep neutral atomic qubits, we use focused laser beams, or rather several interrelated laser beams, which form the so-called optical lattice. Dozens of scientific groups around the world apply this method. Although it is not easy to control neutral atoms and bind them at the level of one qubit, there are several promising ways to do this. As an alternative, many groups use positively charged ions - atoms with one missing electron.
The ions repel each other as a result of the action of electrostatic forces, and they can not be retained in the electrostatic field formed by the electrodes. We can cool with the help of laser radiation hundreds of retained ions in the form of a stationary crystal-like structure of individual atoms that behave like identical pendulums connected by springs. Additional control lasers can swing ions in such a way that their spin states turn out to be quantum entangled as a result of ion oscillations, a scheme first proposed in 1995 by physicists from the Austrian University of Innsbruck. Leopold and Franz Ignacio Cirac and Peter Zoller. Over the past two decades, scientists have made an astonishing breakthrough in the management of individual qubits of trapped ions and their quantum entanglement by this method. Recently, teams led by one of us (Christopher Monroe), David J. Wineland of the National Institute of Standards and Technology and Rainer Blatt from Innsbruck University demonstrated successful experiments on quantum entanglement of 20 qubits on trapped ions.
Scientists have investigated two ways to connect the modules obtained on such quantum-entangled ionic crystals. The first is to physically move several ionic qubits in space from one module to another, forcing them to pass through a complex labyrinth of electrodes (the method proposed in 2000 by Monroe in conjunction with David Kielpinski, then working for the National Institute of Standards and Technology ). The ions can be made to slide through space together with the wave of the electric field so as not to disturb the state of their qubits. When ions touch the second module, the formation of new quantum entanglements can be induced by means of a laser pulse. Two modules, say, 50 qubits each, become part of one computational register, which means that now 100 qubits work together, although loosely connected. Theoretically, there is no limit to the number of modules that can be connected in this way, called the "ion shuttle method".
The difficulty of this method lies in the complexity of managing ingenious ion traps, which consist of hundreds of thousands of precisely located electrodes, through which ions move back and forth. We must be able to manipulate all the necessary voltages on the electrodes to make the ions slide through the labyrinth of these electrodes. Significant efforts to fabricate ion trap electrodes from silicon or other semiconductor materials in a scaling way are applied today at Sandia National Laboratories and at Honeywell.
The second method, which provides the connection of ionic qubit modules, allows atoms to remain in place. In it, in order to force ions to emit photons that are quantum-entangled with ions, lasers are used. These photons can then transfer quantum entanglement between modules. This type of photon quantum interface grows from an idea first voiced almost 20 years ago by scientists from Innsbruck University, Cultech and Harvard University and demonstrated ten years ago by Monroe. The photonic communication technique has a tremendous advantage, allowing us to tie together the qubit memory, the elements of which can be removed a considerable distance, besides it can be applied to another type of qubit, such as neutral atoms, as well as superconducting and semiconductor qubits, which will go below. Moreover, we can scale photonic connections between modules through fiber-optic networks and switches, which will help us to control which of the qubits should be quantum confused. The main obstacle in this way is that the photon coupling of qubits, as a rule, is not effective enough, since it requires capture and accurate direction of these photons. To establish a successful connection, many attempts may be required. The best speed achieved today is the ten quantum entanglements per second. However, the development of the existing technology promises an increase in this speed by several orders of magnitude.
Superconducting qubits
Although atoms can be used as natural qubits, the task of controlling and scaling them into larger systems is fraught with a number of engineering problems. An alternative strategy is to create an "artificial atom" using electrical circuits of superconducting materials. These devices consist of many atoms, but they can behave like simple controlled qubits in which the presence or absence of a single microwave photon or the direction of the current in the circuit clockwise or counterclockwise corresponds to the states "0" or "1". Such quantum circuits have a certain advantage. We can set their properties at the design stage and serialize them with the help of technological processes used in modern microelectronics for the production of conventional integrated circuits. But, what is remarkable, when they work at a temperature close to absolute zero, they can be in the superposition state long enough to serve as a full-fledged qubit. Over the past 15 years, the lifetime of such systems has been increased more than a million times.
In the past decade, in the course of working on superconducting quantum circuits, rapid progress was achieved, demonstrating the various characteristics necessary for a quantum computer. Scientists in many university laboratories, as well as such participants in the high-tech market as Google and IBM, today learned how to manipulate several superconducting qubits and quantum entangle them. With the help of a method called "quantum electrodynamics of electrical circuits", the founder of which was one of us (Robert Shelkopf), together with his colleagues from Yale University Michel H. Devoret (Michel H. Devoret) and Steve Girvin (Steve Girvin), we can even quantum to interchange the qubits at large distances, using superconducting transmission lines.
Superconducting devices naturally allow a modular architecture. We can connect modules and measuring devices inside a large cryogenic device using superconducting conductors and at the same time reduce cross-interference and interference between individual modules by shielding them from each other. To evoke quantum entanglement between modules, scientists at Yale, at OILA of the University of Colorado at Boulder (Joint Institute for Laboratory Astrophysics (OILA), was founded in 1952. Since then, the field of research conducted there has expanded considerably and today, in addition to astrophysics, includes atomic and molecular physics, optics, biophysics, quantum information, precise measurements, and much more, but the acronym remained the same, at the University of California at Berkeley and in other laboratories developed a special superconductor Suitable devices for quantum measurements. The modular approach to superconducting qubits has several attractive features. Instead of building and testing a giant electrical circuit, we only need to produce and calibrate more simple modules in mass quantities, and then complicate the machine by adding a module behind the module.
We can replace or bypass defective modules and re-connect the electrical circuits connecting the modules in order to build another architecture. At present, work is also under way to develop quantum converters that transform the microwave signal into an optical one, then to connect the remote modules with an optical fiber and thus create long-range quantum networks or a distributed quantum computer.
Solid-state spin qubits
Finally, the third type of qubits, in which information is encoded in the form of spin states of solids. There are various models of this type of qubits, but a promising method developed by one of us (Mikhail Lukin), as well as a large number of other groups, uses crystal defects to obtain qubits. One such system is a diamond carbon lattice consisting of carbon atoms, in which one of the carbon atoms is replaced by a nitrogen atom, and the adjacent node is empty, a defect called the "nitrogen-vacancy" center (NV center). Electromagnetic pulses can control the electron spin of this atom-like impurity. In the method first proposed by Lukin and colleagues, the NV center acts on the nuclear spins of its nearest neighbors - carbon atoms, forming a group of neighboring qubits formed as a result of magnetic interaction between the particles. However, the number of carbon atoms-the nearest neighbors of the nitrogen-vacancy defect-can be counted on the fingers, which limits the total number of qubits per module by less than a dozen.
The scaling problem requires the connection of a plurality of modules together. If the qubits are located in different crystal lattices, we can bind them, forcing each qubit to emit a photon, and then to measure the photons. If several NV-centers are located inside one diamond lattice, we can also try to establish a connection between them, using quantum oscillations, called phonons, which can transfer quantum information between impurities.
It is noteworthy that, although manipulation of the information encoded in the qubits of these NV-centers is extremely difficult, we can often do all this under normal conditions at room temperature. The methods of observing a single NV center, proposed in the past decade by Jörg Wrachtrup of the University of Stuttgart and Fedor Zhelezko, currently working at the University of Ulma (Fedor Borisovich Zhelezko, graduate of the Belarusian State University, after his defense in 1998 Candidate's dissertation emigrated to Germany, now - Director of the Institute of Quantum Optics at the University of Ulm, allowed scientists to work with individual qubits of electronic spins. The team led by David Awschalom of the University of Chicago managed to manipulate these qubits on a nanosecond scale, which is comparable to the speed of modern classical processors.
Recently at the Dutch Delft University of Technology, Ronald Hanson (Ronald Hanson) and his colleagues have confused the quantum of qubits of single NV impurities distant from each other over a distance of more than one kilometer, using quantum entangled photons, similar to the photon method that allows to bind ions, which was described earlier . While this process is not very effective (in the Delft experiment, quantum entanglement links are established at a rate of only a few times per hour), but recently at Harvard University and at the Massachusetts Institute of Technology invented new methods using nanooptical devices that make it possible to accelerate it to a large extent. And since we already have tools that allow us to form a few qubits around a single diamond lattice defect and store them for more than a second in ultra-pure crystals, such as those grown by Element Six, NV centers show great potential for the scalable modular architecture of a quantum computer.
Quantum future
As a result of more than 20 years of research and development in this field, scientists have experimentally tested all the approaches described above to a modular quantum computer on a small scale. The task that awaits us is to extend these methods to larger conglomerations of qubits and modules and start using them for interesting applications. We believe that today this goal is just around the corner.
A quantum future is both tempting and challenging. As quantum machines become more and more, manage them and control the fact that, on the whole, the system really behaves quantum-mechanically, it will become increasingly difficult. Fortunately, the modular architecture allows us to test and evaluate the operation of individual modules and various connections between them independently of each other, without disrupting the operation of the whole system. Scientists have recently taken important steps towards this goal.
But modular quantum computers, even on a relatively modest scale, are likely to enable us to solve unique problems. They naturally become the backbone of the "quantum Internet", consisting of small quantum processors, combined with the help of quantum-entangled optical photons. They can serve as repeaters that extend the geographical scope of secure quantum communication systems (currently limited to about 100 km, since photons attenuate in standard fiber-optic lines) to continental distances.
Elements of modular quantum machines are already beginning to be included in some of the most accurate chronometers in the world, and their role is expected to grow in a new generation of optical atomic clocks based on neutral atoms and ions. Scientists have proposed to build a global quantum network of such watches to create a single international time scale, or, roughly speaking, a "world clock" that will work with unprecedented stability and accuracy.
A miniature quantum network could also serve as a high-precision sensor of electromagnetic fields and temperature in complex chemical and biological systems on a nanometer scale. For example, scientists have used electronic and nuclear spins associated with impurities in solids to achieve magnetic resonance imaging with resolution in a single atom. This method can probably be used for direct observation of individual molecules, which will give an unusually powerful tool of fundamental biology and materials science, as well as new means of medical diagnostics and the search for new drugs.
It's time to stop asking if a quantum computer is possible, and start focusing on developing its large-scale architecture and what it can do. The truth is that no one knows how quantum computers will change our world. But with the advent of the era of networks of modular quantum computers, we were on the verge of change.

References:
1, 2, 3, and 4
Support @steemstem and the #steemstem
project - curating and supporting quality STEM
related content on Steemit




Congratulations @punjolife, this post is the seventh most rewarded post (based on pending payouts) in the last 12 hours written by a Newbie account holder (accounts that hold between 0.01 and 0.1 Mega Vests). The total number of posts by newbie account holders during this period was 3809 and the total pending payments to posts in this category was $3215.71. To see the full list of highest paid posts across all accounts categories, click here.
If you do not wish to receive these messages in future, please reply stop to this comment.