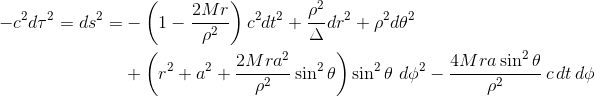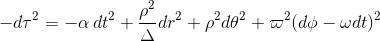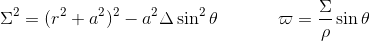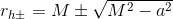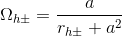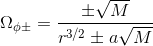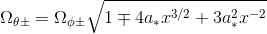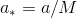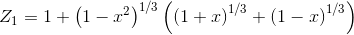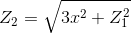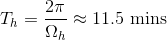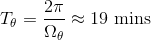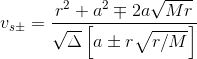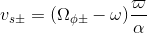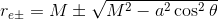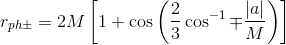Calculating the Spin of Sagittarius A*, the Supermassive Black Hole at the Centre of our Galaxy
Spin and mass of the nearest supermassive black hole by V I Dokuchaev
https://arxiv.org/abs/1306.2033
Extracts from the abstract-
'Quasi-Periodic Oscillations (QPOs) of the hot plasma spots or clumps orbiting an accreting black hole contain information on the black hole mass and spin. The promising observational signatures for the measurement of black hole mass and spin are the latitudinal oscillation frequency of the bright spots in the accretion flow and the frequency of black hole event horizon rotation... Interpretation of the known QPO data by dint of a signal modulation from the hot spots in the accreting plasma reveals the Kerr metric rotation parameter, a=0.65 ± 0.05, and mass, M=(4.2 ± 0.2)106 M⊙, of the supermassive black hole in the Galactic center. At the same time, the observed 11.5 min QPO period is identified with a period of the black hole event horizon rotation, and, respectively, the 19 min period is identified with a latitudinal oscillation period of hot spots in the accretion flow..'
I was interested that this paper calculated the quantity of spin for the black hole at the centre of our galaxy, which is nearly 26,000 light years from Earth, using a handful of observations and calculations (note, the mass is calculated from the orbits of nearby stars). While the equations themselves are fairly simple, it's worth first taking a look at Kerr metric (which deals with the curvature of spacetime due to gravity and spin) from which they are derived-
One of the most common expressions for Kerr metric is-
where  and
and 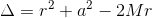 . τ is proper (or local) time, s is spacetime and t is coordinate (or at infinity) time, M is the gravitational radius (a geometric unit for mass) where M=Gm/c2 and a is the spin parameter (a geometric unit of spin) where a=J/mc.
. τ is proper (or local) time, s is spacetime and t is coordinate (or at infinity) time, M is the gravitational radius (a geometric unit for mass) where M=Gm/c2 and a is the spin parameter (a geometric unit of spin) where a=J/mc.
This can also be written in a condensed form (and with c=1) as-
where angular velocity of the frame dragging rate (ω) which is the rate that spacetime is dragged around the black hole as observed from infinity and the reduction factor (α) are given by-
Note, multiply ω by c for SI units of rad/s. The reduction factor (or lapse function) can be described as the redshift and time dilation at a specific r taking into account dilation due to gravity and frame dragging (for instance, the frame dragging rate as observed from infinity would be ω but the proper frame dragging rate locally would be ω/α). Other geometric functions are given by-
where ϖ is often referred to as the reduced circumference (or the proper coordinate radius that takes into account frame dragging).
The black holes event horizons (outer and inner) are given by-
where + defines the outer horizon and − defines the inner.
Angular velocity of the event horizons (as observed from infinity)-
where again, + defines the outer and − defines the inner horizon. Angular velocity (in its most simple form) required for a stable equatorial orbit at a specific r is given by-
Here, + defines a prograde orbit (with the frame dragging) and − the retrograde orbit (against the frame dragging). Allowing for resonance (the rate at which an object in orbit bobs up and down in latitude due to the highly curved spacetime), the angular velocity with latitudinal oscillation of a near circular orbit at a specific r is given by-
This angular velocity describes the latitudinal oscillation of the hot spot or clump of plasma in the thin accretion disk (Note, Ωθ grows slightly less than ΩΦ the closer r is to the black hole, the orbit velocity is probably the same but due to slight movements in latitude, the angular velocity for Ωθ is less than ΩΦ which is a mathematical exact equatorial orbit , see fig. 6 in the paper). It is assumed that the brightest hot spots in the accretion flow are located near the inner edge of the accretion disk, corresponding to the radius of the marginally stable circular orbit given by-
where
The marginally stable orbit is at 6M for a static black hole and reduces the greater the spin is, down to M for a hypothetical maximal black hole where a=M, hence the spin parameter can be deduced from where the marginally stable orbit resides.
Based on Quasi-Periodic Oscillations (QPOs) of ~11.5 minutes at the event horizon (Th) and ~19 minutes at the marginally stable orbit (Tθ), the following would apply (considering only the outer event horizon and the prograde orbit) -
where rms would be used to calculate Ωθ. In both cases, the spin parameter is calculated to be a/M≈0.65 in order to match the orbit periods (T), as per fig. 8 in the paper. (Note, the equation for T is based on circumference/velocity where circ=2πϖ and v=Ωϖ where ϖ replaces what would normally be r).
Other useful calculations from Kerr metric-
Proper velocity required for a stable orbit in the equatorial plane (where + defines a prograde orbit and − the retrograde orbit)-
which is equivalent to-
Ergoregion boundaries (where + defines the outer and − defines the inner boundary, the inner residing within the inner horizon)-
Photon sphere (or more accurately, the photon circle in the equatorial plane, where + defines the prograde and − defines the retrograde orbit), 3M for a static black hole-
Marginally bound circular orbit (where orbits are unstable), 4M for a static black hole-