Bose-Einstein Condensates

Introduction 

Bose-Einstein condensates, are in my opinion some of the most amazing, and awe-inspiring phenomena that we, as a species are aware of. Their bizarre and recondite properties are direct results of quantum mechanics. In essence, they are macroscopic quantum phenomena, and can only be observed at very low temperatures. The principal discoveries have taken place over the course of a hundred years, starting in the early years of the twentieth century and still continuing with great pace, to this day! 

The field of low temperature physics can be said to have had its beginnings in 1908, when helium was first liquified at the laboratory of H. Hammerling Onnes in Leiden, The Netherlands. It wasn't terribly along afterwards when superconductivity was discovered in the same laboratory! Despite this long history, research into these states of matter is still developing, and has been completely revolutionised with novel discoveries in recent years. Over the years we have progressed to study systens at lower and lower temperatures, with the current cutting edge characterised by the study of atomic BEC at nano-Kelvin temperatures. 

---

 

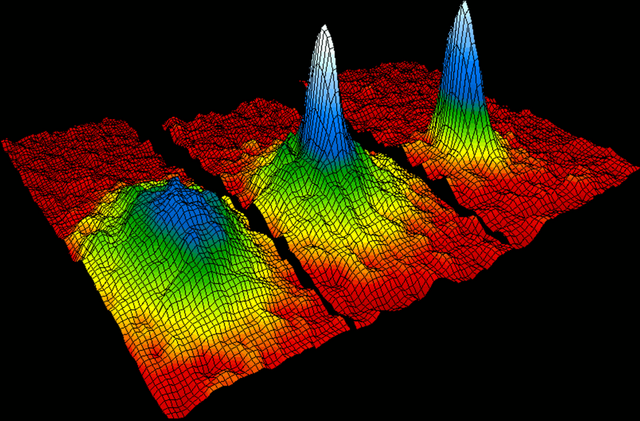
Successive occurrence of Bose-Einstein condensation in rubidium. From left to right is shown the atomic distribution in the cloud just prior to condensation, at the start of condensation and after full condensation. High peaks correspond to a large number of atoms. Silhouettes of the expanding atom cloud were recorded 6 ms after switching off the confining forces of the atom trap. 

 [ source/image credit ]

---

Historical perspective 

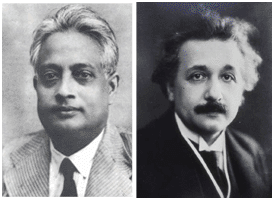	In 1924, Bose wrote to Einstein describing his new method to derive the Planck black-body formula. At that time Einstein was already a superstar, having just won the Nobel prize for his groundbreaking work on the photoelectric effect.

Bose was a relatively unknown scientist, at the time, working in Bangladesh (then, Dacca). His previous letters to European journals had been ignored, but Einstein saw the potential in his novel ideas and helped him to publish the results. 

The idea that Bose introduced was to treat the electromagnetic waves of the block-body as a gas of identical particles. This was the first time that the spooky light quanta (introduced by Planck and Einstein to account for the photoelectric effect) could be thought of as particles! Yes - particles of light; we now refer to them as photons! 

Einstein extended these ideas to the regime of an ideal gas of particles, with non-zero mass. This was the first fully fledged quantum mechanical generalisation of the standard classical theory of the ideal gas. We know now that there are two distinct quantum ideal gases, corresponding to either Bose-Einstein or Fermi-Dirac statistics. The method of counting quantum states introduced by Bose and Einstein applies to boson particles, such as photons or ⁴He atoms.  The main idea, here, is that for identical quantum particles, we can simply count the number of available quantum states using combinatorics. 

---

Bose-Einstein statistics

If we have N_s identical Bose particles in M_s available quantum states then there are 

 

available ways that the particles can be distributed. Now, we apply this combinatoric rule to the thermodynamics of an ideal gas of N boson particles which are occupying some volume V. Using periodic boundary conditions, it is the case that any individual atom will be in a plane-wave quantum state, 

 

where the allowed wave vectors are  

 

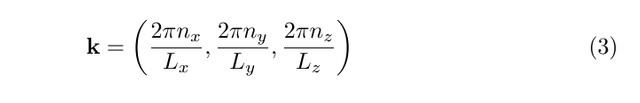
and where L_x, L_y, and L_z are the lengths of the volume in the x, y and z directions. The total volume is given by V = L_xL_yL_z, and thus an infinitesimal volume d³k = dk_xdk_ydk_z of k-space contains 

 

quantum states. Each of these single particle quantum states has an energy  

 

where m is the particle mass. The next step is a clever trick of dividing up the available single particle quantum states into a number of thin spherical shells of states. By equation (5), a shell of radius k_s and thickness δk_s contains 

 

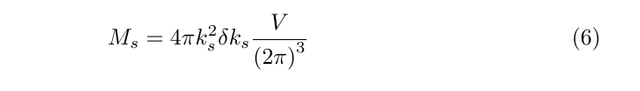
single particle states. 

The number of available states between energy  ε_s and  ε_s + δε_s is therefore 

 

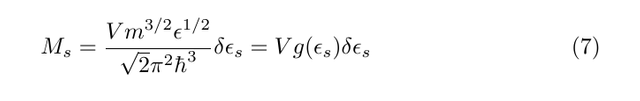
where g(ε) is the density of states per unit volume. We know from the fundamentals of statistical mechanics that the total entropy of the gas is given by S = k_B ln W, where k_B is Boltzmann's constant and W is the number of available microstates of a given total energy E. Using the Stirling approximation, and writing the total number of available microstates for the whole gas as the product of the number of available states in each k-space shell, it is possible to show that the number of particles in each energy shell, N_s, is given by  

 

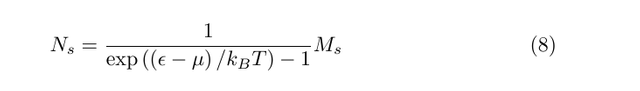
Now, the average number of particles occupying any single quantum state is N_s/M_s, so the average occupation number of any given single particle state of energy ε_k is given by the Bose-Einstein distribution 

   

---

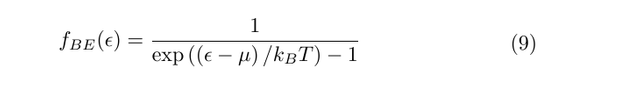
Over the course of the next few posts on BECs, we will examine the consequences of this result, and consider BEC in ultra-cold atomic gases. Cheers, D.