Problem solving strategies - Lesson 5
Problem solving strategies:
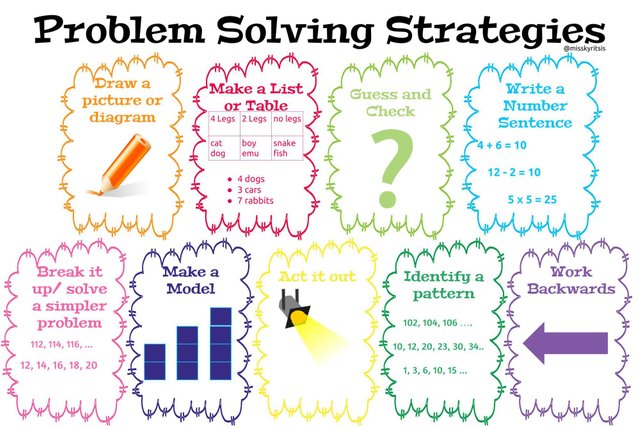
- Look for a pattern
- Make an organized list
- Draw a table
- Guess and check (Trial and improvement)
- Work backwards
- Use logical reasoning
- Draw a diagram
- Solve a simpler problem
Now that we have had a look at the previous 7 problem solving strategies, it brings us to the last lesson. In this lesson we are going to have a look at solving a simpler problem.
Example:
There are 24 teams in a netball league. Each of these 24 teams, has to play each other twice during the season. How many matches will take place in a season?

Make the problem simpler by working with fewer teams first.
We can use 3 teams as a simpler problem:

Teams: A , B , C
We can also take these 3 teams so that each one plays against the other teams twice.
The matches will take place as follow: AB , AC, BC which is 3 matches.
We can see that each team only plays against another team 2 times.
If these matches had to be played twice, there will be 6 matches during the season.
Therefore: 3 teams x 2 matches = 6 matches

If there are 4 teams: A , B , C , D, the matches will take place as follow:AB , AC , AD , BC , BD , CD which is 6 matches.
If each match occurred twice, there will be 12 matches in total.
We can see that the teams plays against each other 3 times.
Therefore, 4 teams x 3 matches = 12 matches in total
If we had 5 teams, we can say that these teams will play 4 matches each.
Therefore, 5 teams x 4 matches = 20 matches in total.
Now that we have a calculation for a simpler sum, we can calculate the actual question.
24 teams, will play 23 matches. Therefore, 24 teams x 23 matches = 552 matches.
During the season, 552 netball matches will be played.
Activity:
Mr. Johnson wants to organize a knockout tournament for soccer. There are 14 teams in the tournament. How many matches will have to be played until the winner is found?
(NOTE: In a knockout tournament, the winners go to the next round, while the losers are knocked out of the tournament.)
Remember: There may be more than one way to solve a problem. The strategies discussed in this lesson as well as all the previous lessons are all just examples of how a problem can be solved.
Mathematics can be solved by using a lot of different strategies of problem solving or methods. That is what makes mathematics so much fun, there are always more than one way to calculate sums.

Hmm.You theory is welcoming. There are many ways to solve a problem.So also there are may problem for every one solve.
So you see.How can you balanxe this and bring it to equilibrum.
Challenges always pomping up. No wonder persons quit
Am not disputing your theory.Infact is a great and immense help.
Thank you
Hi @joshuaky, yes in Mathematics there are different ways in solving problems. As a teacher, you have to be the one encouraging learners to never give up, but rather to find a method or strategy that will work for them. We can teach all we want, but studens have to give their cooperation too. It is easier to just give up, that's why we are there to motivate them in finding easier solutions.
Dear @apteacher, I love your class today, math is very important, because with them we can solve activities that have to do with solving real life problems that may be present. Thank you for your wisdom, God bless you.
Regards ...
Thank you for the comment. Mathematics is very important indeed!! Especially in solving real life problems😁
You have been upvoted by the @sndbox-alpha! Our curation team is currently formed by @jeffbernst, @bitrocker2020, @jrswab & @teachblogger . We are seeking posts of the highest quality and we deem your endeavour as one of them. If you want to get to know more, feel free to check our blog.
You got a 2.58% upvote from @postpromoter courtesy of @apteacher!
Want to promote your posts too? Check out the Steem Bot Tracker website for more info. If you would like to support the development of @postpromoter and the bot tracker please vote for @yabapmatt for witness!