Sophism #11: The Liar's Paradox
The Liar's Paradox is perhaps the shortest sophism there is. In its most common form it fits in just four words:
This sentence is false.
Think about it: if the sentence is indeed false, then we should not believe its claim that "it is false", and must conclude that the sentence is, in fact, truthful. However if it is truthful, then we must believe its claim that it is false, and thus must conclude that it is false, returning back to where we started. It is an infinite loop!
The liar's paradox is the father of all logical sophisms. Despite its deceivingly compact form, it bears profound implications for various corners of logic, mathematics and computing. For example, its construction is mirrored in the Russel's paradox, which lies at the foundation of modern set and category theory (and thus, indirectly, all of modern mathematics).
The liar's paradox is also to blame for the existence of the halting problem and Rice's theorem. These two severely curb the ambitions of computer science by demonstrating that it is mathematically impossible to create an algorithm which could reliably detect any useful properties of other algorithms.
The paradox achieves full glory within the famous Gödel's First Incompleteness Theorem, which, in simple terms, claims that in any reasonably complex logical system (such as our mathematics) there will always exist statements which are impossible to either prove or disprove. A version of the "liar's paradox sentence" is essentially an example of such a statement.
The liar's paradox can be cast in many different forms. The one with the two boxes is among my favourites:
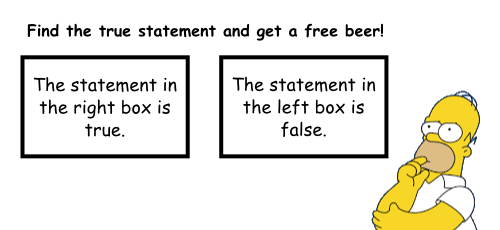
For other sophisms check out my other posts.
What's great about Godel's First Incompleteness Theorem is that "reasonably complex logical system" (I think...) just means that you have to be able to do arithmetic! That's pretty basic in my mind...and it just makes the statement even more mind-blowing! Nice post!
When we speak about formal axiomatic systems, "just being able to do arithmetic" is not that "basic", really.
The thing is, there are infinitely many different natural numbers, and the ability to represent natural numbers along with some meaningful operations on them (which, by the way, requires at least 8-9 axioms, which is, in some sense, a lot) gives the formal system access to this "power of infinity". The power of infinity is not something to be taken lightly :)
In fact, you might notice at some point that among the sophisms that I post here, all of the "really tricky ones" (i.e. not those which are based on a camouflaged mistake) are in some sense related to infinity.
Congratulations @konstantint! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP