THE SUM OF THE INTERIOR ANGLES OF EVERY TRIANGLE IS 180°

I want to show you that the angles A plus B plus C is always 180°. Of course, this applies to Euclidean geometry, which is the geometry everyone uses. Non-Euclidean geometry is generally only used by high level mathematicians; so this demonstration is valid for virtually every triangle you would ever use in math or in life if you are a reader of this post. The demonstration for this is quite easy and can help you with your math.
First note that a straight line is 180°. This can either be determined by definition of a straight angle or from a definition of a circular angle as 360°.

Secondly, take the bottom line of the triangle and make a parallel to it. Place the parallel above the base as shown next. Then draw a single line crosswise through these two parallel lines. The crosswise line is named the transversal. It will be the left side of the our triangle in the present case.
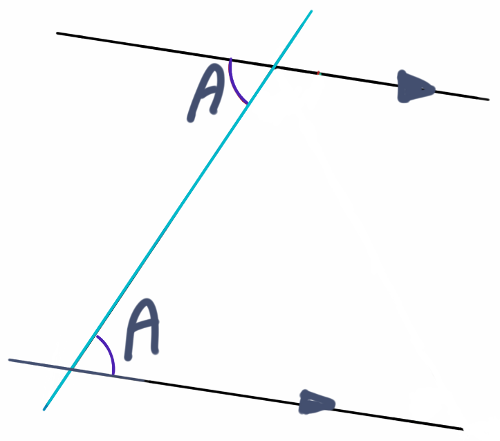
The alternate interior angles labeled A are congruent; they represent the same angle measure. This is true for all transversals cutting through a set of parallel lines. If you would like me to demonstrate this fact in a different post, please let me know.
Once recognized, the sum of the angles of a triangle is solved. Let’s place that parallel line through the vertex at angle B as shown below.

In consideration of the triangle, the internal angle A is the same as the alternate interior angle which is adjacent to angle B on the left; the internal angle C is the same as the alternate interior angle which is adjacent to angle B on the right. The straight angle at the top is formed by the angles A, B, and C. This is 180° as already mentioned.
Thus,
A+ B + C = 180°
This is what I wanted to demonstrate.