Mathematics : Geometry of Transformation at Batik Art In Indonesia

Dear Steemian Friends. i will share about Geometry of Transformation in Batik Art.

Geometry in Batik

geometry transformation is widely used in real life, as well as in making batik. in Indonesia is very famous for batik. apparently in the manufacture of batik art also uses the science of geometry tansformasi

Batik is an art work of Indonesia's cultural heritage. The beauty of batik has been recognized by the world through the establishment of UNESCO since October 2, 2009 that batik is one of the humanitarian heritage for the work of oral and non material (Masterpieces of the Oral and Intangible Heritage of Humanity). Batik art is not only dominated by Javanese culture, because other regions in Indonesia also have a painting of cloth (if so called) or batik. High artistic painting can be found on cloth ornaments of ulos (batak), sasirangan (South Kalimantan), or from other parts of Indonesia namely batik Papua, Sulawesi batik and so on. This shows how rich our culture is.

In this case we can conclude that batik is the work of our ancestral relics. in this case the way of making batik is related to the science of transformation geometry. many other things are made that also use the science of transformation geometry.

The beauty of batik can be enjoyed from the artistic forms are poured on the sheet of cloth. When carefully observed, in the forms of batik there are actually rhythmic or patterned patterns of order. Some forms of regularity in batik is a formation of geometric transformation.

Geometric shapes that can be found in batik in the form of dots, lines and plane. Flat fields such as circles, ellipses, rectangles and so on. Artistic formation in batik is produced through the transformation of point, line or plane through translation, shift, reflection or dilation.

Application of Reflection (Reflection) on Batik Motif

Here is one of Kawung batik motif.

Image Source

The basic shape is the ellipse and the point (Fig 1).

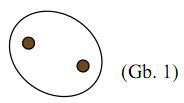
Image Source

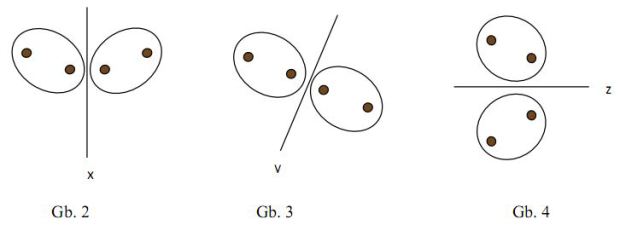
The formation on kawung batik motif can be seen as the result of reflection (reflection) the basic form. The result of reflection of image 1 on the lines x, y, and z gives the following form orientation (Figs. 2, Figs .3, and Fig.4).

()
Image Source

Combined figures 1, 2, 3, and 4 produce a formation in the following kawung batik (Figure 5)

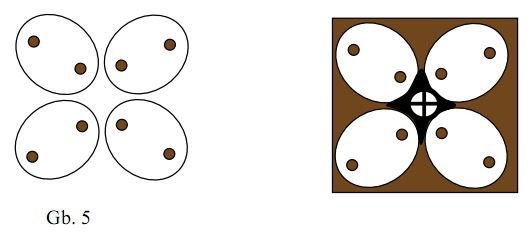
Image Source

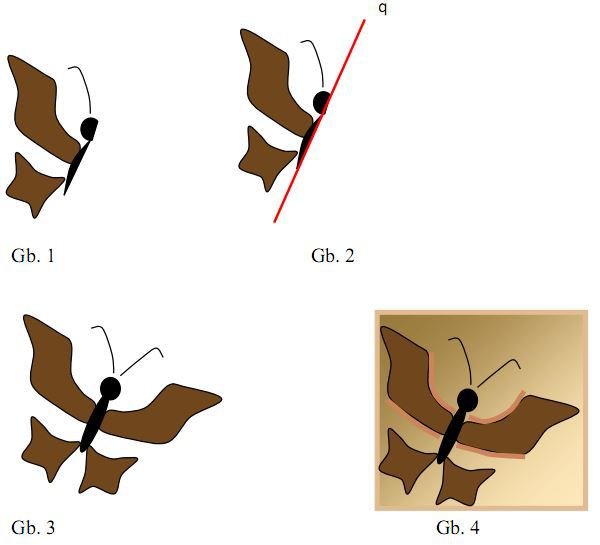
Here is one of Madura batik motif.

Note the shape of the butterfly on the Madura batik motif. The shape of the butterfly is symmetrical, so it can be viewed as a result of reflecting some of the wake-ups against the symmetry axis.
Its basic shape is a curved line and some form of wake flat (Fig 1). Then it is reflected on the symmetry axis of the line q (Fig.2), to obtain the intact form of a butterfly (Figure 3).

Image Source

Application Rotation (Turnover) on Batik Motif

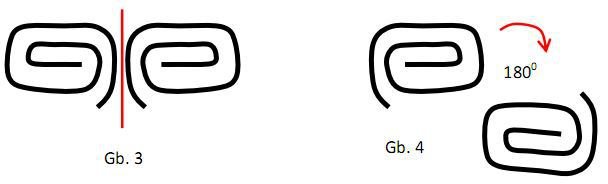
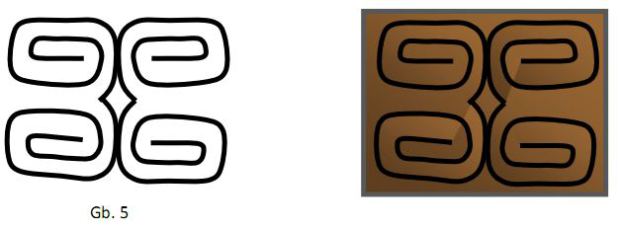
Here is one of the motifs of Papuan batik.

Image Source

The basic form of the Papuan batik motif is the curved line (Figure 1)

Image Source

Furthermore, the basic shape is rotated 180 degrees (Fig.2)

Image Source

Other forms are obtained by reflection of the vertical line (Fig. 3) and then rotated 180 degrees (Fig.4)

Image Source

Combined from Figures 1, 2, 3, and 4 produce the following form of Papuan batik motif (Fig.5)

Image Source

Translational Application (Shift) on Batik Motif

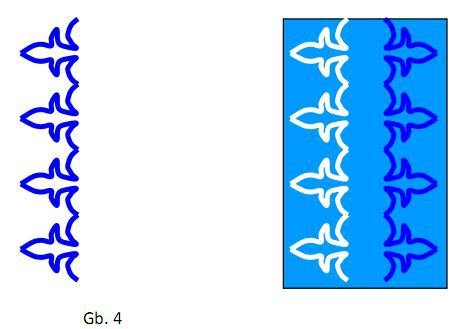
Here is one of the sasirangan motifs (Borneo) called the waves sinampar coral.

Image Source

The basic form of this sasirangan motif is a curved line (Fig 1).

Image Source

Furthermore, the incorporation of the basic shape reflection (Fig.1) to the horizontal line produces a petal-like shape (Fig. 2).

Image Source

Suppose that the petal-like motifs are placed on the axis of cartesius, then the shape of the petals is subsequently obtained by the following or vector translation T_(1=(■(0@-b))) shift (Figure 3).

Image Source

And so on the translation is done by using the vector formula T_(n=(■(0@-nb))) with n is the original number so obtained the series of flower petals that form the wave motive of the following coral sinampar (Fig. 4).

Image Source

Application Dilation (Multiplication) on Batik Motif

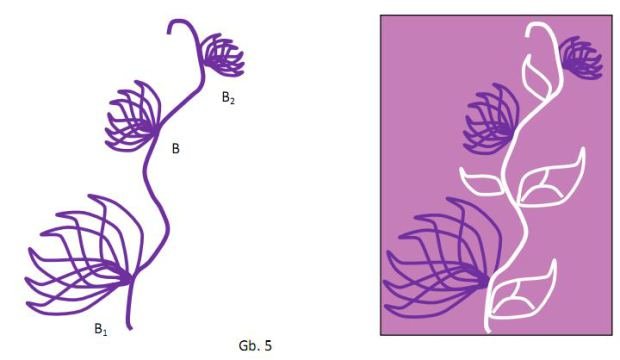
Here is one of the kasiung kaumbakan sasirangan motifs.

Image Source

Notice the lotus-like motif on the sasirangan. The basic shape of the lotus flower is a flat wake (Fig 1) which can be viewed as a lotus petal, then through several rotations and reflections obtained by the arrangement of petals to form the lotus (Fig. 2).

Image Source

The lotus flower painted on kangkung kaumbakan motif above has a different size, where the size or magnitude of the flower can be viewed as the result of dilation or multiplication by a constant k to image form 2 where k is the real number of posituf. Furthermore, the shape of Figure 2 is referred to as B.
Let k1 = 2, then the form k1B is magnification twice B, let's call the result k1B = B1 (Fig.3).
Then to obtain the shape of the next lotus by taking k2 = 1/3, call it
result k2B = B2 (Fig.4).

Image Source

To obtain the artistic position on the stalk, then B2 is reflected on the vertical line so that the arrangement is made to form kumbung kaumbakan motif (Fig.5).

So are some examples of applications of transformation geometry in batik artwork in Indonesia. Pattern shape on batik motifs can be an alternative source of learning mathematics for our students. In addition to students acquiring knowledge related to the concept of transformation geometry, they also understand the application of transformational geometry that can produce works of art. In line with this, through the investigation of the pattern of batik motifs, students are expected to appreciate the art of his own people so that the love of the country grows.

*REFERENCE

• Wikepedi.html

Image Source

Thanks For Visiting My Blog @Nafazul,,,Don't Forget Follow, Upvote and Resteem