Sketching in a curved space #1

The story..

Urban Sketching is one of the most amazing activity one can do in the summer. Fortunately, I live in a very old city in France, with stoned streets and churches everywhere.

Drawing a church is a challenge, unless you can fix a good angle from an helicopter or some kind of drone. Obviously, this is not may case. I am pedestrian an only my imagination can fly. But, my imagination never surrender, never. And she often chats with my mathematical brain, they have a lot of fun. So my imagination asked to the mathematician inside of me, if it is possible to draw 180º at a street level, with this angle, we can have a nice print of the church. My insight mathematician, turn his head lifting his eyebrow and murmured:

-Of course, but not in an Euclidean Geometry.
-What do you mean? -answered my imagination.
-You have to curve the space -Replied my mathematician.
-Curve the space? -my imagination was surprised.
-Aha, curve the space -stated my mathematician
-You mean, curve the space in the way Einstein did with the Cartesian space? replied my imagination. (It is important to notice that my imagination listens carefully to my mathematician, when she has the occasion, his words are dense and spare, he is terrible old man taking pleasure studying math works from Bernhard Riemann, Einstein, Gauss and company, so she heard from Einstein curvature of the space)
-So, if we curve the space, both vanishing points at the left and the right of the head will be connected by a curve? replied my imagination, excited.
-Yes, young lady. -replied my mathematician.
-And what curve is it? -asked, curious, my imagination.
-It's a geodesic in a sphere, pretty obvious.. whispered my mathematician.
-Geodesics in a sphere! mm, that must be a sexy curve! exclaimed my imagination.
-They are, I confirm. -replied my mathematician.
-Could you draw one for me?
-There are different ways to do this. You can start with the Hamilton's minimal action principle, then calculate Christoffel Symbols for a sphere and integrate the ordinary differential equation. Or you can assume a parallel transport of a particle walking through the sphere, assuming no variation in norm and direction. Both alternatives get the same result.
-Christoffel Symbols! I love this powerful Gamma (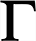)! Looks so greek! I love when you show me your equations full of greek letters!
-hmm... - answered the mathematician.

Then the mathematician build a nice geodesic grid and flattened it into a square, like this:

()

Spherical Geodesic Grid

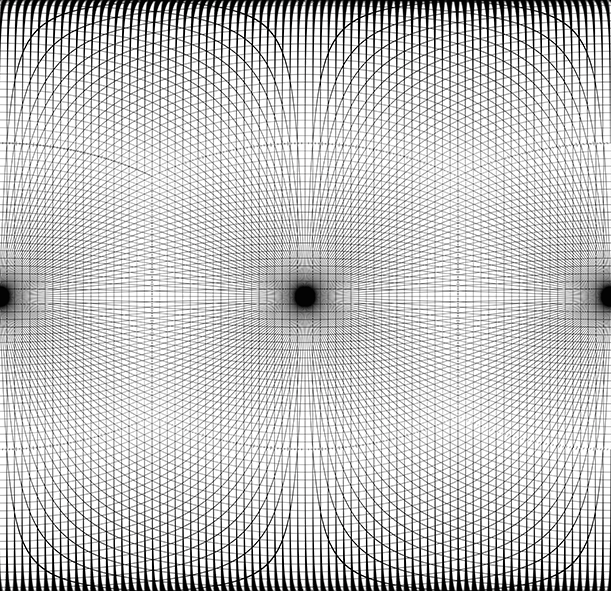
My imagination, then, sat down, opened her notebook and carefully forwarded the visual information following the grid geodesics. To express the beauty of the lines she decided to chose a fountain pen with flexible nib. She add some nice lights and shades, add some textures to imitate the stone walls and roads. And finally she drew the mathematician and herself at the bottom right corner of the sketch. It took her no more than 30 minutes.

The final result..

Imagination and Mathematician, a summer allegory in Angoulême, France