26-10-2024 - Education - Geometry - basis of a vector space [EN]-[IT]

~~~ La versione in italiano inizia subito dopo la versione in inglese ~~~
[ENGLISH]
26-10-2024 - Education - Geometry - basis of a vector space [EN]-[IT]
With this post I would like to provide some brief notions about the technical topic mentioned in the subject.
The context in which we operate is that of analytical geometry.
(code notes: MOD-90)
Basis of a vector space
Considerations:
Completing a basis in analytical geometry means starting from a set of linearly independent vectors in a vector space and adding other vectors.
The main concept is the following.
A basis of a vector space V of dimension n is a set of n linearly independent vectors that generate V.
A set of vectors is expressed in the following way.
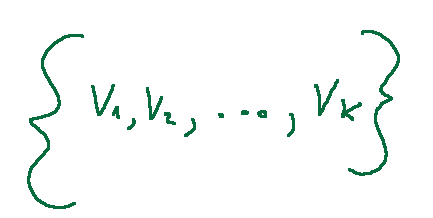
To complete a basis in a vector space of dimension n we must perform the following steps:
1-Verify linear independence
2-Determine how many vectors are missing
3-Find the additional vectors
4-Verify the independence of the added vectors
5-Build the complete basis.
Example
Let's take the following set of vectors as an example

Let's go over the 5 points described before
1-Verify linear independence
Here we note that there is linear independence between these two vectors, there is no factor that multiplied by one of the two vectors generates the other.
2-Determine how many vectors are missing
The set is R4 so it has a base of 4 elements, while here we only have 2, so 2 vectors are missing.
3-Find the additional vectors
Here we can use the matrix method or the method of reduction to the scale form.
Example
Suppose we have the following two vectors
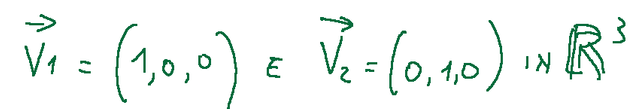
These vectors are linearly independent but they do not form a complete basis in R3, because the space has dimension 3 but we have only two vectors.
To complete the basis we can add the following vector V3, which is a vector independent of the other two written above.
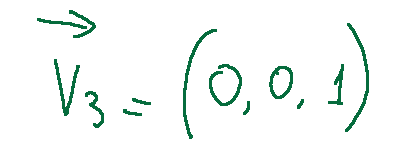
So now we can have the set R3 completely defined.
So the basis of R3 will be the following.
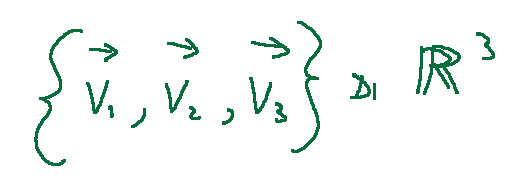
So taking into consideration the set given before, that is the set R4, we can say that two vectors are missing to complete the basis and we can obtain 2 independent vectors that can be the following (written in red)
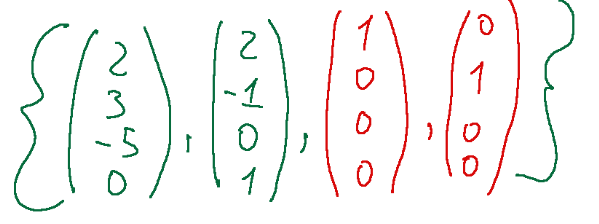
4-Verify the independence of the added vectors
The added vectors are independent of the first two since there are no coefficients that multiplied by the first vectors can generate the added vectors
5-Build the complete basis.
Here is the complete basis
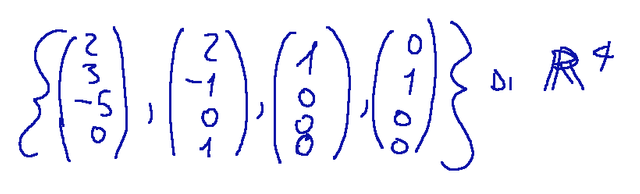
Conclusions
In analytical geometry, a basis is an ordered set of linearly independent vectors that generate a vector space V.
Question
Did you study vector spaces at school?

[ITALIAN]
26-10-2024 - Education - Geometria - base di uno spazio vettoriale [EN]-[IT]
Con questo post vorrei fornire alcune brevi nozioni a riguardo dell’argomento tecnico citato in oggetto.
Il contesto in cui operiamo è quello delle geometria analitica.
(code notes: MOD-90)
Base di uno spazio vettoriale
Considerazioni:
Completare una base in geometria analitica significa partire da un insieme di vettori linearmente indipendenti in uno spazio vettoriale e aggiungere altri vettori.
Il concetto principale è il seguente.
Una base di uno spazio vettoriale V di dimensione n è un insieme di n vettori linearmente indipendenti che generano V.
Un insieme di vettori si esprime nella seguente maniera.
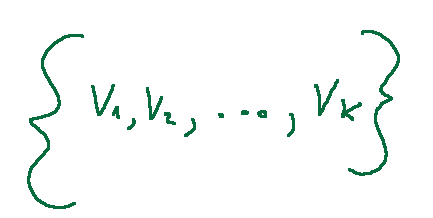
per completare una base in uno spazio vettoriale di dimensione n dobbiamo eseguire i seguenti passaggi:
1-Verificare l’indipendenza lineare
2-Determinare quanti vettori mancano
3-Trovare i vettori aggiuntivi
4-Verificare l’indipendenza dei vettori aggiunti
5-Costruire la base completa.
Esempio
Prendiamo ad esempio il seguente insieme di vettori

Ripercorriamo i 5 punti descritti prima
1-Verificare l’indipendenza lineare
Qui notiamo che tra questi due vettori c’è indipendenza lineare, non c’è un fattore che moltiplicato ad uno dei due vettori generi l’altro.
2-Determinare quanti vettori mancano
L’insieme è R4 quindi ha una base di 4 elementi, mentre qui ne abbiamo solo 2, quindi mancano 2 vettori.
3-Trovare i vettori aggiuntivi
Qui possiamo usare il metodo della matrice o il metodo della riduzione alla forma di scala.
Esempio
Supponiamo di avere i seguenti due vettori
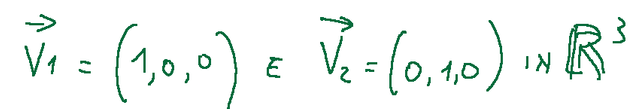
Questi vettori sono linearmente indipendenti ma non formano una base in R3 completa, perché lo spazio ha dimensione 3 ma noi abbiamo solo due vettori.
Per completare la base possiamo aggiungere il seguente vettore V3, che è un vettore indipendente dagli altri due scritti sopra.
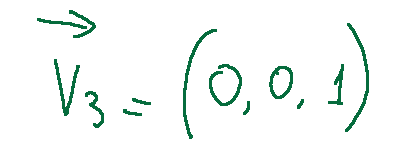
Così ora possiamo avere l’insieme R3 completamente definito.
Quindi la base di R3 sarà la seguente.
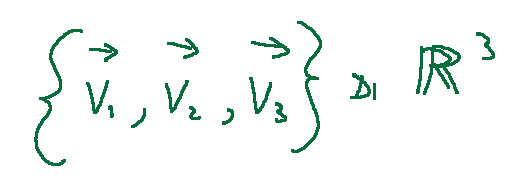
Quindi prendendo in considerazione l’insieme dato prima, cioè l’insieme R4, possiamo dire che mancano due vettori per completare la base e possiamo ricavare 2 vettori indipendenti che possono essere i seguenti (scritti in rosso)
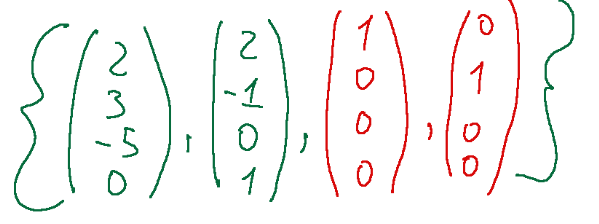
4-Verificare l’indipendenza dei vettori aggiunti
I vettori aggiunti sono indipendente dai primi due in quanto non ci sono coefficienti che moltiplicati ai primi vettori possano generare i vettori aggiunti
5-Costruire la base completa.
Qui di seguito la base completa
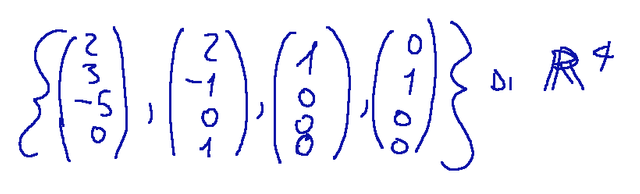
Conclusioni
In geometria analitica, una base è un insieme ordinato di vettori linearmente indipendenti che generano uno spazio vettoriale V.
Domanda
A scuola avete studiato gli spazi vettoriali?
THE END
Ricordo questo .
Ti auguro un buon inizio settimana
I love the way you are actually able to explain this in details that I was actually able to pick a lot of things from it. This makes me to remember those period when one was studying physics
🤖 Ehi @stefano.massari! 💃 Robot dance mode in onore di questo post! Sono il bot di @luciojolly, nato per brillare! 📚